Chemical reactions
Rodrigo Canisella Fávero
Paper and oxygen rearranging themselves into $CO_2$ and water (vapor), giving off heat.
Conserving atoms
Dalton thought that...
Many substances can be "decomposed" into other substances, as a result of some sort of chemical reaction. (E.g. heating, electrolysis, or even mechanical grinding). We call these substances compounds.
- But there are some substances which cannot be decomposed further. These are the elements. An element consists of just one kind of atom.
- No atoms are ever created or destroyed in these processes. Chemical reactions are re-arrangements of molecules (consisting of atoms) into other molecules (consisting of the same atoms).
Since atoms are never created or destroyed, we say that atoms are conserved in any chemical reaction
Decomposition
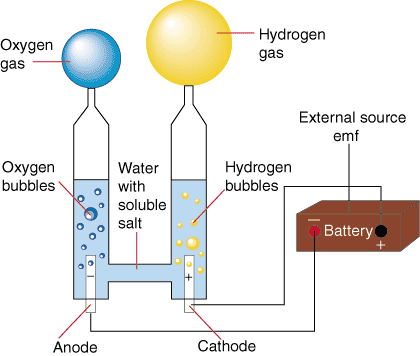
Electrolysis of water:
We describe this situation with a chemical reaction equation:
$$2H_2O \rightarrow 2H_2 + {\color{#bbb}1}O_2$$
Chemical balance
Left side of the reaction: $2 H_2O$
2 molecules of $H_2O$. Each molecule has 2 atoms of H and 1 atom of O.
Total 4 atoms of hydrogen and 2 atoms of oxygen.
Right side of the reaction: $2 H_2 + O_2$
2 molecules of $H_2$ contain 4 atoms of H.
1 molecule of $O_2$ contains 2 atoms of oxygen.
Often you are in the situation of know what chemicals you start with (the "reactants"), and what chemicals you end up with (the "products"). To balance a chemical reaction you must figure out how many of each kind of molecule you need to make the number of each kind of atom come out the same on both sides.
Balance these chemical reactions (they are all "combustion" reactions, in which something burns by combining with oxygen)
Coal is solid carbon, $C$. show / hide
$$
1C +
\text{__}O_2 \rightarrow \text{__}CO_2$$
$$
1C +
\color{blue}
\text{_1_}
\color{black}
O_2 \rightarrow \color{blue}\text{_1_}\color{black}CO_2$$
That is, 1 Carbon atom, 2 Oxygen atoms on both sides.
This is usually written without the ones, as:
$
C +
O_2 \rightarrow CO_2$
Ethane show / hide
$$2C_2H_6 +\text{__}O_2 \rightarrow \text{__}CO_2 + \text{__}H_2O$$
Hint: How many $C$ atoms and $H$ atoms are there on the left? Next, work out how many molecules you need on the right to balance the $C$ and $H$ from the left. Then come back to the oxygen on the left.
The only molecule on the left with carbon or hydrogen is Ethane, $C_2H_6$, and we're starting with 2 of those. In one molecule of ethane there are 2 $C$ atoms and ___ $H$ atoms. So in two molecules there must be 4 $C$ atoms and ___ $H$ atoms. That's all the $C$ and $H$ on the left.
Switching to the right side of the equation: the only molecule with any $C$ is $CO_2$ with one atom of $C$ per molecule. To balance the 4 $C$'s on the left we must have four molecules of $CO_2$. Similarly, ___ $H_2O$ molecules would be needed to have ___ $H$ atoms.
$$\text{_2_}C_2H_6 +
\text{_?_}O_2 \rightarrow \text{_4_}CO_2 + \text{_6_}H_2O$$
Now, we can figure out how many atoms of $O$ on the right. the 4 $CO_2$ molecules have $4\times 2=8$ $O's$. and the 6 $H_2O$ molecules have 6 $O$'s. So that's $6+8=14$ O's on the right.
Looking at the left side, we only have $O_2$ molecules, with 2 $O$'s each. So 7 molecules of $O_2$ will have 14 atoms on the left. The final, balanced equation is:
$$\text{2}C_2H_6 +\color{blue}
\text{7}\color{black}O_2 \rightarrow \color{blue}\text{4}\color{black}CO_2 + \color{blue}\text{6}\color{black}H_2O.$$
It would also be true to write:
$$\text{_1_}C_2H_6 +\frac 72 O_2 \rightarrow \text{_2_}CO_2 + \text{_3_}H_2O$$
This is fine as far as I'm concerned! Later, when we use this in combustion, you'll get the right answers when we calculate.
But some chemists want there to be *only integer* numbers in front of each molecule. To satisfy these folks, you can just multiply *all* the numbers of molecules by 2 (if you get any number with 2 in the denominator).
Methane (natural gas) show / hide
$$1CH_4 +\text{__}O_2 \rightarrow \text{__}CO_2 + \text{__}H_2O$$
$$\text{_1_}CH_4 +\color{blue}\text{_2_}O_2\color{black} \rightarrow \color{blue}\text{_1_}\color{black}CO_2 + \color{blue}\text{_2_}\color{black}H_2O$$
4 $H$ and 4 $O$ and 1 $C$ on each side.
Ethanol (alcohol) show / hide
$${\color{#bbb}1}C_2H_6O +\text{__}O_2 \rightarrow \text{__}CO_2 + \text{__}H_2O$$
$$\text{_1_}C_2H_6O +\color{blue}\text{_3_}\color{black}O_2 \rightarrow \color{blue}\text{_2_}\color{black}CO_2 + \color{blue}\text{_3_}\color{black}H_2O$$
7 $O$, 2 $C$, and 6 $H$ on each side.
Octane (one of the main molecules in gasoline) show / hide
$$2C_8H_{18} + \text{___}O_2 \to \text{___}CO_2 + \text{___}H_2O$$
$$2C_8H_{18} + \text{25}O_2 \to \text{16}CO_2 +\text{18}H_2O
$$